CHAPTER 5
Chapter 5 - Differentiating Functions
Section 5.1 -
Differentiating
Functions
Differentiation is the process of
finding the rate of change of a function. We have proven that if f is a variable dependent on an
independent variable x, such that
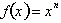then
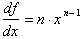where n is a positive
integer. The derivative reflects the instantaneous rate of change of the
function at any value x. The
derivative is also a function of x
whose value is dependent on x.
Take a look at the left side of the
function,
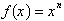By definition the
derivative of a dependent variable, f,
is
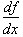, which is the instantaneous rate
of change of
f with respect to x at any condition x. The
right side of the function,
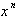, represents the independent variable whose derivative is
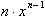
When differentiating a function of
the form
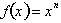, the derivative of the dependent variable is,
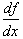, and the derivative of the independent variable is
. Thus differentiating a function results in a new function
of x, where
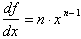. The derivative is called
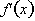, read “f prime of x”, and it represents the derivative of
a function of x with respect to the independent
variable, x.. If
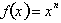, then:
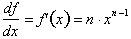
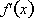gives the
instantaneous rate of change of f(x)
as a function of any value, x. Remember
that the rate of change of a function other than a line is not constant. Its
value changes as x changes.
If f(x) were equal to a constant multiplied by a function of x such
as:
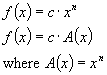
The derivative
of f(x) would be:
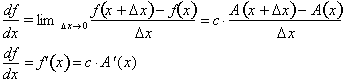
Thus the derivative of f(x) with respect to x, is the constant multiplied by the
derivative of the function of x, A(x).
Next section ->
Section 5.2 - Differentiating Sums of Functions
