CHAPTER 7 - Study of Free Falling Bodies
Chapter 7 - Study of Free Falling Bodies
Section 7.1 - What is a Force?
An object in motion is
characterized by a changing position as a function of time. The derivative of
the distance function with respect to time gives us the velocity of
the object as a function of time. Furthermore the derivative of the
velocity function, gives us the acceleration of the object as a function of
time. But what causes an object to move? To understand particle dynamics we
need to first understand the concept of force.
Newton's first law of motion states that an object in motion will
remain in motion until acted on by a force.
This observation is one of most important ones ever made as it offers a way of
defining what a force is. According to this law, an object traveling in space
at 100,000 km/hr will remain at that velocity forever provided no force acts on
it.
For example, if you were to throw a ball in space it
would forever continue in the same direction
along with the same velocity with which it left your hand. Here, space refers to anywhere that is free
from the influence of any gravitational force, electro-magnetic force,
air-resistance or any other forces. Once set in motion an object will continue
with that same velocity forever.
Since no force is required to keep an object
in motion, then a force can be defined as that which changes the velocity of the object. Thus force is a measure of a
resistance to a change in motion. Since motion is characterized by a constant velocity, then a change in motion
results in a change in velocity. By definition, a change in velocity is an acceleration.
This simple, yet
profound conclusion tells us that forces are defined by accelerations. The
force required to accelerate an object is proportional to the magnitude of the
acceleration. The mass of the object is also a factor since the greater the
mass, the greater its resistance to motion. Observation shows that the resistance to a change in
motion is directly dependent on the amount of matter being accelerated.
We can define a
Newton as the force equipped accelerate a body of unit mass, 1 kg,
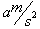Therefore to accelerate a body of mass, m, the
required force would be m times a.
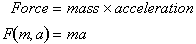
This is read as, the force required to accelerate a body
is directly related to its mass and the magnitude of the acceleration of the mass. The important concept to understand
is that forces are defined as accelerations or changes in velocity. It requires no force to keep a body in motion
Once in motion it will remain in motion. A force is required only to change its
velocity or accelerate it. Thus force
is a quantifiable measurement of a mass’s resistance to a change in motion. If
an object had no resistance to a change in motion then there would be no such
thing as force!
This might seem to contradict reason. One can better
understand this by considering an airplane flying in space, where space is some
imaginary place that contains no matter or force fields inside it. If its four
engines produce an acceleration
and the mass of the
plane is
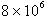kg, then the thrust
or force acting on the plane is
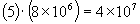. If we assume an inexhaustible and weightless fuel source
then theoretically the engines will push the plane forward with a constant force of 4 million newtons.
Now since the plane is flying in an imaginary space under
a constant force, it is free to accelerate forever. Remember forces are defined
as accelerations and not velocities. The plane will accelerate at a constant acceleration of
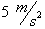. This means the planes velocity
would increases and increase at the rate of
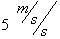The graph of its velocity as a function of time would be a linearly increasing
function:
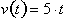
The derivative of the
velocity function is the acceleration function:
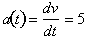
The fundamental concept to understand here is that a
force is required only to change an
objects velocity. A change in velocity is by definition an acceleration.
Therefore forces are required only to accelerate an object. A constant force
acting on a body will accelerate the body with a constant acceleration, which
means the body’s velocity will increase and increase forever, all due to a constant force. Furthermore the greater the mass, the greater its
resistance to a change in velocity. Thus, the force required to accelerate a
mass is directly proportional to its mass.
Next section ->
Section 7.2 - Understanding Free-Fall Motion
