CHAPTER 6 - Applications of the Derivative
Chapter 6 - Applications of the Derivative
Section 6.1 - Motion, Velocity and Acceleration
We have developed the essential theory for defining and
analyzing the mathematical function. The derivative of a function was defined
as the instantaneous rate of change
of a function at any given point or moment.
Geometrically the derivative was simply the slope of the tangent to the
graph at a particular point.
As a student you are probably still a little confused by
the concept of instantaneous rate of change. To strengthen your
understanding of the derivative, let us
scientifically analyze a physical phenomena and see how exactly the derivative
is defined for the situation. The
situation we will study is that of the dynamic motion of a body.
Motion is characterized by a changing distance between a
reference point and the object itself. Distance is measured in the dimension of
length with units of meters, miles, or feet. We know how to measure distance
but how do we measure a changing distance? Whenever a dimension changes with
respect to itself it constitutes an action
that defines time. Thus a changing distance has to be measured relative to a
change in time.
We can define velocity to be a measure of how fast an
object in motion moves. Thus velocity is the rate at which the distance is
changing relative to time. A simpler definition is that velocity is the
distance covered per unit time. If an object moves 100 m in one second, then
its velocity for that interval is
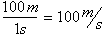. The units of velocity are meters per second or change in distance per change in unit time. Consider a for a sports car moving at a constant
velocity of 100
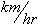. If we plot graph distance covered from a reference point,
we get.
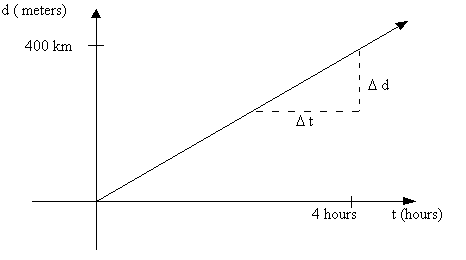
The graph is a linearly increasing straight line, where
the distance covered increases directly with time. The steepness or rate of
change of this graph is by definition the change in distance over the change in
time:
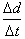
Since motion is characterized by a
change in position relative to time, then velocity is only defined over that
definite time interval. If an object moves from a to b, the velocity of the
object over this interval is this interval
distance covered,
divided by the time taken to cover this
distance,
.
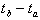Thus, velocity is the rate of change of distance covered with respect to time:
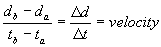
For example, the car above
takes one hour to go from a station 400
kilometers down the highway to another station 500 kilometers down the same
highway. The velocity of the car is then
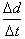
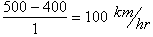
Since the graph of the car’s distance covered with
respect to time is a straight line, it has a constant rate of change, such that
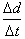= v, where v is a constant velocity. This tells us that the
velocity over the entire journey is a constant or
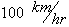. The graph of this velocity function is just a straight
line, or is the same at any time t.
This confirms logic since throughout the journey the
speedometer reads a constant 100 km./hr. Therefore, distance covered is directly related to the elapsed time,
in each hour it will cover a 100 more kilometers.
Now let us define acceleration. Acceleration is the rate
of change of velocity with respect to time. Acceleration is to velocity as
velocity is to distance. The concept of acceleration refers to a changing
velocity per unit time. The units of acceleration are
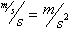
For example if the acceleration of a Ferrari is 4 km/hr
per second, all this means is that each second the velocity increases or changes
by 4 km/hr. If the velocity at t=3 seconds is 48 km/hr, then the velocity
at t=6 seconds will be:
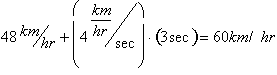
Lets see how this applies to a car moving with constant
acceleration. Since the acceleration is constant, the graph is a horizontal
line, where the acceleration of the car is at any time t is the same.
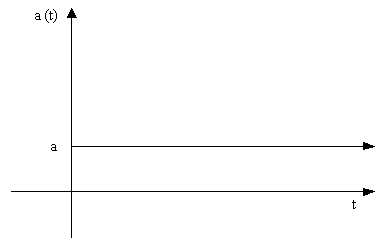
The graph of
velocity as a function of time,
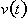will increase directly with time for a car
moving with constant acceleration.
Acceleration is therefore the rate of change of velocity with respect to time or
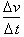. An acceleration of 4 m/s/s means that every second the velocity increases by 4 m/s.
The concept of acceleration and velocity are fairly
obvious to understand when dealing with constant accelerations and velocities.
We now need to define a more precise way of explaining velocities as the derivative of the position or distance
function with respect to time and acceleration as the derivative of the velocity function with respect to time.
Next section ->
Section 6.2 - Instantaneous Velocity and Acceleration
